Poker Hand Odds Four Of A Kind
POKER PROBABILITIES
- Texas Hold'em Poker
Texas Hold'em Poker probabilities - Omaha Poker
Omaha Poker probabilities - 5 Card Poker
5 Card Poker probabilities
In poker, the probability of each type of 5-card hand can be computed by calculating the proportion of hands of that type among all possible hands. Frequency of 5-card poker hands The following enumerates the (absolute) frequency of each hand, given all combinations of 5 cards randomly drawn from a full deck of 52 without replacement.
POKER CALCULATOR
- Poker calculator
Poker odds calculator
POKER INFORMATION
- Poker hand rankings
Ranking of poker hands
In poker, the probability of each type of 5-card hand can be computed by calculating the proportion of hands of that type among all possible hands.
Frequency of 5-card poker hands
The following enumerates the (absolute) frequency of each hand, given all combinations of 5 cards randomly drawn from a full deck of 52 without replacement. Wild cards are not considered. The probability of drawing a given hand is calculated by dividing the number of ways of drawing the hand by the total number of 5-card hands (the sample space, five-card hands). The odds are defined as the ratio (1/p) - 1 : 1, where p is the probability. Note that the cumulative column contains the probability of being dealt that hand or any of the hands ranked higher than it. (The frequencies given are exact; the probabilities and odds are approximate.)
The nCr function on most scientific calculators can be used to calculate hand frequencies; entering nCr with 52 and 5, for example, yields as above.
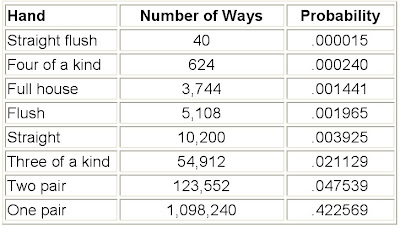
| Hand | Frequency | Approx. Probability | Approx. Cumulative | Approx. Odds | Mathematical expression of absolute frequency |
|---|---|---|---|---|---|
| Royal flush | 4 | 0.000154% | 0.000154% | 649,739 : 1 | |
| Straight flush (excluding royal flush) | 36 | 0.00139% | 0.00154% | 72,192.33 : 1 | |
| Four of a kind | 624 | 0.0240% | 0.0256% | 4,164 : 1 | |
| Full house | 3,744 | 0.144% | 0.170% | 693.2 : 1 | |
| Flush (excluding royal flush and straight flush) | 5,108 | 0.197% | 0.367% | 507.8 : 1 | |
| Straight (excluding royal flush and straight flush) | 10,200 | 0.392% | 0.76% | 253.8 : 1 | |
| Three of a kind | 54,912 | 2.11% | 2.87% | 46.3 : 1 | |
| Two pair | 123,552 | 4.75% | 7.62% | 20.03 : 1 | |
| One pair | 1,098,240 | 42.3% | 49.9% | 1.36 : 1 | |
| No pair / High card | 1,302,540 | 50.1% | 100% | .995 : 1 | |
| Total | 2,598,960 | 100% | 100% | 1 : 1 |
The royal flush is a case of the straight flush. It can be formed 4 ways (one for each suit), giving it a probability of 0.000154% and odds of 649,739 : 1.
When ace-low straights and ace-low straight flushes are not counted, the probabilities of each are reduced: straights and straight flushes each become 9/10 as common as they otherwise would be. The 4 missed straight flushes become flushes and the 1,020 missed straights become no pair.
Note that since suits have no relative value in poker, two hands can be considered identical if one hand can be transformed into the other by swapping suits. For example, the hand 3♣ 7♣ 8♣ Q♠ A♠ is identical to 3♦ 7♦ 8♦ Q♥ A♥ because replacing all of the clubs in the first hand with diamonds and all of the spades with hearts produces the second hand. So eliminating identical hands that ignore relative suit values, there are only 134,459 distinct hands.
The number of distinct poker hands is even smaller. For example, 3♣ 7♣ 8♣ Q♠ A♠ and 3♦ 7♣ 8♦ Q♥ A♥ are not identical hands when just ignoring suit assignments because one hand has three suits, while the other hand has only two—that difference could affect the relative value of each hand when there are more cards to come. However, even though the hands are not identical from that perspective, they still form equivalent poker hands because each hand is an A-Q-8-7-3 high card hand. There are 7,462 distinct poker hands.
Derivation of frequencies of 5-card poker hands
of the binomial coefficients and their interpretation as the number of ways of choosing elements from a given set. See also: sample space and event (probability theory).
- Straight flush — Each straight flush is uniquely determined by its highest ranking card; and these ranks go from 5 (A-2-3-4-5) up to A (10-J-Q-K-A) in each of the 4 suits. Thus, the total number of straight flushes is:
- Royal straight flush — A royal straight flush is a subset of all straight flushes in which the ace is the highest card (ie 10-J-Q-K-A in any of the four suits). Thus, the total number of royal straight flushes is
- or simply . Note: this means that the total number of non-Royal straight flushes is 36.
- Royal straight flush — A royal straight flush is a subset of all straight flushes in which the ace is the highest card (ie 10-J-Q-K-A in any of the four suits). Thus, the total number of royal straight flushes is
- Four of a kind — Any one of the thirteen ranks can form the four of a kind by selecting all four of the suits in that rank. The final card can have any one of the twelve remaining ranks, and any suit. Thus, the total number of four-of-a-kinds is:
- Full house — The full house comprises a triple (three of a kind) and a pair. The triple can be any one of the thirteen ranks, and consists of three of the four suits. The pair can be any one of the remaining twelve ranks, and consists of two of the four suits. Thus, the total number of full houses is:
- Flush — The flush contains any five of the thirteen ranks, all of which belong to one of the four suits, minus the 40 straight flushes. Thus, the total number of flushes is:
- Straight — The straight consists of any one of the ten possible sequences of five consecutive cards, from 5-4-3-2-A to A-K-Q-J-10. Each of these five cards can have any one of the four suits. Finally, as with the flush, the 40 straight flushes must be excluded, giving:
- Three of a kind — Any of the thirteen ranks can form the three of a kind, which can contain any three of the four suits. The remaining two cards can have any two of the remaining twelve ranks, and each can have any of the four suits. Thus, the total number of three-of-a-kinds is:
- Two pair — The pairs can have any two of the thirteen ranks, and each pair can have two of the four suits. The final card can have any one of the eleven remaining ranks, and any suit. Thus, the total number of two-pairs is:
- Pair — The pair can have any one of the thirteen ranks, and any two of the four suits. The remaining three cards can have any three of the remaining twelve ranks, and each can have any of the four suits. Thus, the total number of pair hands is:
- No pair — A no-pair hand contains five of the thirteen ranks, discounting the ten possible straights, and each card can have any of the four suits, discounting the four possible flushes. Alternatively, a no-pair hand is any hand that does not fall into one of the above categories; that is, any way to choose five out of 52 cards, discounting all of the above hands. Thus, the total number of no-pair hands is:
- Any five card poker hand — The total number of five card hands that can be drawn from a deck of cards is found using a combination selecting five cards, in any order where n refers to the number of items that can be selected and r to the sample size; the '!' is the factorial operator:
This guide is licensed under the GNU Free Documentation License. It uses material from the Wikipedia.
Home > 5 Card Poker probabilities
Wizard Recommends
- €1500 Welcome Bonus
- €100 + 300 Free Spins
- 100% Welcome Bonus
On This Page
Introduction
Four Poker is a new poker variation invented by Roger Snow and marketed by Shufflemaster. The game is similar to Three Card Poker but as the title suggests, four cards are used instead of three. Also, there is no dealer qualifying hand and the player can raise up to three times his ante. However, the dealer gets one extra card to form his best hand.
Rules
- Two initial bets are available: The Ante and the Aces Up.
- All players get five cards each and the dealer gets six cards. One of the dealer cards is placed face up, and five face down.
- Players making the Ante bet must decide to fold or raise.
- If the player folds he forfeits his Ante bet. He may or may not forfeit his Aces Up bet, depending on casino rules. It shouldn't matter because if the player has a paying Aces Up bet, he shouldn't be folding anyway.
- If player raises, then he must raise at least the amount of the Ante and at most, three times the Ante.
- The player keeps his best four cards and discards one.
- Following is the ranking of hands from lowest to highest: high card, pair, two pair, straight, flush, three of a kind, straight flush, four of a kind.
- After all decisions have been made, the dealer will turn over his cards and select the best four out of six.
- The player's hand shall be compared to the dealer's hand, the higher hand winning.
- If the dealer's hand is higher, then the player shall lose the Ante and Raise.
- If the player's hand is higher or equal then the Ante and Raise shall pay one to one.
- If the player has at least a three of a kind, then he shall also be paid a Bonus, regardless of the value of the dealer's hand. Two different pay tables are available for the Bonus, as displayed below, and are based on the ante bet. Pay Table 1 is the only one I know of to be actually used.
- Another bet is available (similar to the Pairplus in Three Card Poker), based only on the player's four card hand, called the Aces Up. Seven pay tables are available as indicated below. The only one I know of to be actually used is pay table 5.
Bonus Pay Table
| Hand | Table 1 | Table 2 |
|---|---|---|
| Four of a kind | 25 | 30 |
| Straight flush | 20 | 15 |
| Three of a kind | 2 | 2 |
Aces Up Pay Table
| Hand | Table 1 | Table 2 | Table 3 | Table 4 | Table 5 | Table 6 | Table 7 |
|---|---|---|---|---|---|---|---|
| Four of a kind | 50 to 1 | 50 to 1 | 50 to 1 | 50 to 1 | 50 to 1 | 50 to 1 | 50 to 1 |
| Straight flush | 40 to 1 | 40 to 1 | 30 to 1 | 30 to 1 | 40 to 1 | 40 to 1 | 40 to 1 |
| Three of a kind | 9 to 1 | 7 to 1 | 9 to 1 | 7 to 1 | 8 to 1 | 8 to 1 | 7 to 1 |
| Flush | 6 to 1 | 6 to 1 | 6 to 1 | 6 to 1 | 5 to 1 | 6 to 1 | 5 to 1 |
| Straight | 4 to 1 | 5 to 1 | 4 to 1 | 5 to 1 | 4 to 1 | 4 to 1 | 4 to 1 |
| Two pair | 2 to 1 | 2 to 1 | 2 to 1 | 2 to 1 | 3 to 1 | 2 to 1 | 3 to 1 |
| Pair of aces or better | 1 to 1 | 1 to 1 | 1 to 1 | 1 to 1 | 1 to 1 | 1 to 1 | 1 to 1 |
Of these pay tables for the Aces Up side bet, number five is the most popular. The only exceptions that I'm aware of are an unconfirmed report that that Tulalip in Washington uses pay table 4 and the Grand Casino Hinckley in Minnesota uses pay table 1.
Analysis
The following return table is based on optimal player strategy under the 2-20-25 Ante Bonus pay table. The lower right cell shows a house edge of 2.79%.
Return Table Based on Optimal Strategy
| Player Hand | Raise/Fold | Win/Loss | Combinations | Probability | Pays | Return |
|---|---|---|---|---|---|---|
| Four of a Kind | 3 | Win | 40,182,878,736 | 0.000240 | +29 | 0.006960 |
| Four of a Kind | 3 | Lose | 18,594,576 | 0.000000 | +21 | 0.000002 |
| Straight Flush | 3 | Win | 133,224,330,456 | 0.000796 | +24 | 0.019096 |
| Straight Flush | 3 | Lose | 265,177,080 | 0.000002 | +16 | 0.000025 |
| Three of a Kind | 3 | Win | 3,675,379,352,400 | 0.021951 | +6 | 0.131703 |
| Three of a Kind | 3 | Lose | 103,559,138,928 | 0.000618 | -2 | -0.001237 |
| Flush | 3 | Win | 6,599,621,152,728 | 0.039415 | +4 | 0.157660 |
| Flush | 3 | Lose | 784,564,849,080 | 0.004686 | -4 | -0.018743 |
| Straight | 3 | Win | 5,257,469,039,688 | 0.031399 | +4 | 0.125597 |
| Straight | 3 | Lose | 1,301,555,952,216 | 0.007773 | -4 | -0.031093 |
| Two Pair | 3 | Win | 5,539,444,298,496 | 0.033083 | +4 | 0.132333 |
| Two Pair | 3 | Lose | 2,420,447,417,280 | 0.014456 | -4 | -0.057823 |
| One Pair | 3 | Win | 14,764,551,298,548 | 0.088179 | +4 | 0.352714 |
| One Pair | 3 | Lose | 10,806,299,820,804 | 0.064539 | -4 | -0.258155 |
| One Pair | 1 | Win | 13,535,004,289,296 | 0.080835 | +2 | 0.161671 |
| One Pair | 1 | Lose | 22,887,448,286,136 | 0.136691 | -2 | -0.273382 |
| One Pair | Fold | Fold | 5,495,692,732,992 | 0.032822 | -1 | -0.032822 |
| High Card | 1 | Win | 148,058,445,132 | 0.000884 | +2 | 0.001769 |
| High Card | 1 | Lose | 422,493,233,796 | 0.002523 | -2 | -0.005047 |
| High Card | Fold | Fold | 73,523,856,056,112 | 0.439108 | -1 | -0.439108 |
| Totals | 167,439,136,344,480 | 1.000000 | -0.027879 |
The average final bet under optimal strategy is 2.142342 units, making the element of risk, -0.027879/2.142342 = 1.30%. The standard deviation, relative to the original bet, is 2.71.
Beginner Strategy
A simple strategy to this game, first proposed by Stanley Ko, is as follows.
- Raise 3X with a pair of tens or higher.
- Raise 1X with a pair of twos to nines.
- Fold all other.
According to the second edition of 'Beyond Counting' by James Grosjean, this 'simple strategy' results in a house edge of 3.396%.
Intermediate Strategy
The following intermediate strategy was created to balance power and simplicity by our own JB.
- Pair of Aces or better: Bet 3X
- Pair of Js, Qs, Ks: Bet 3X if dealer's upcard is lower than your pair or matches a rank in your hand, otherwise bet 1X
- Pair of 9s, 10s: Bet 1X if dealer's upcard outranks your pair, otherwise bet 3X
- Pair of 8s: Bet 3X if dealer's upcard is a 2, otherwise bet 1X
- Pair of 3s, 4s, 5s, 6s, 7s: Bet 1X
- Pair of 2s or AKQ: Bet 1X if dealer's upcard matches a rank in your hand, otherwise fold
- All other: Fold
Against the 2-20-25 Ante Bonus pay table, the house edge is 2.8526% and the element of risk is 1.3233%.
Advanced Strategy
I'm proud to present the following advanced strategy, also created by my sidekick JB.
- Pair of Aces or better: Bet 3X
- Pair of Ks: Bet 3X, except bet 1X against an Ace and you don't have an Ace nor 4.
- Pair of Js or Qs: Bet 3X, except bet 1X if the dealer's card outranks pair your pair rank and does not match a singleton in your hand.
- Pair of 9s or 10s: Bet 3X, except bet 1X if dealer card outranks your pair rank.
- Pair of 8s: Bet 1X, except bet 3X against a 2
- Pair of 4s thru 7s: Bet 1X
- Pair of 3s: Bet 1X, except fold against a Jack if your highest kicker is a 10 or lower
- Pair of 2s or AKQ: Fold, except bet 1X if dealer card matches a rank in your hand
- AKJT: Fold, except bet 1X against a Jack
- AKJ9 or lower: Fold
Against the 2-20-25 Ante Bonus pay table, the house edge is 2.8498% and the element of risk is 1.3216%. Here is a house edge comparison of various known strategies.
- Simple: 3.396%
- Intermediate: 2.853%
- Advanced: 2.850%
- Optimal: 2.788%
To put it another way, here are the cost of errors:
- Simple: 0.606%
- Intermediate: 0.065%
- Advanced: 0.062%
- Optimal: 0.000%
Aces Up Analysis
The next table shows the probability of each hand and the return under pay table five of the Aces Up side bet. The lower right cell shows a house edge of 3.89%.
Return for Aces Up Pay Table 5
| Hand | Combinations | Probability | Pays | Return |
|---|---|---|---|---|
| Four of a kind | 624 | 0.00024 | 50 | 0.012005 |
| Straight flush | 2072 | 0.000797 | 40 | 0.03189 |
| Three of a kind | 58656 | 0.022569 | 8 | 0.180552 |
| Flush | 114616 | 0.044101 | 5 | 0.220504 |
| Straight | 101808 | 0.039173 | 4 | 0.15669 |
| Two pair | 123552 | 0.047539 | 3 | 0.142617 |
| Pair of aces | 81096 | 0.031203 | 1 | 0.031203 |
| Nothing | 2116536 | 0.814378 | -1 | -0.814378 |
| Total | 2598960 | 1 | -0.038917 |
The next table shows the house edge according to all four Aces Up pay tables.
Aces Up House Edge
| Pay Table | House Edge |
|---|---|
| 1 | 1.98% |
| 2 | 2.58% |
| 3 | 2.78% |
| 4 | 3.37% |
| 5 | 3.89% |
| 6 | 4.24% |
| 7 | 6.15% |
Poker Odds Of Making Hands
Note: There is also a similar game called Crazy Four Poker.
Acknowledgments
I would like to recognize:
- JB for the analysis of the optimal strategy.
- Stanley Ko for the simplified strategy.
- James Grosjean for the unpublished advanced strategy.